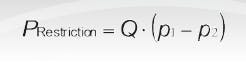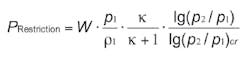Considering Control Valve Efficiency

DOWNLOAD a PDF of this article as it appeared in Flow Control magazine:

The discussions revolving around the scarcity of fossil fuels and increasing energy prices have refocused on the most effective action in the long term, i.e., reducing energy consumption. The right process automation technology can contribute to achieving considerable energy savings.

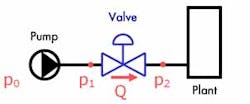
Fluid System Consisting of Pump, Control Valve & a Plant

In processing plants, media or fluids flow through processing equipment. These flows are controlled to reach certain reaction temperatures or pressures at different locations in the plant. The simplest setup of a fluid system includes a pump (or compressor), a control valve, and plant components, such as pipelines, instruments, tanks, etc. (Figure 1).

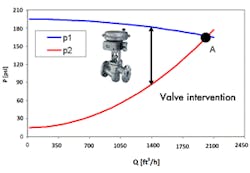
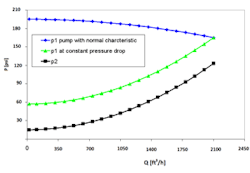
Due to reaction pressures and plant losses, a pump (or compressor) is required to get the flow to move through the plant by creating a pressure differential. The control valve acts as a final element that influences process variables like pressure, temperature and flowrate. It is controlled by the process control system for the control task at hand and intervenes by changing the fluid flow in the system. It bridges the gap between the flowrate Q, which is changed by the pump, and the plant pressure (p₁ and p₂). Without a control valve, the flowrate (Q_Plant) would be as indicated by point A in Figure 2.

Figure 1. Fluid system consisting of pump, control valve, and a plant

Pressures p₁ and p₂ differ when the flowrate is smaller than Q_Plant. At a certain flowrate Q, p₁ is determined by the pump characteristic and p₂ by the plant characteristic. The valve’s throttling effect must be adapted using the variable Cv coefficient, which expresses the flow capacity, and thus the associated valve opening.

Figure 2. Pump plant characteristic

It is often said that control valves wastefully expend energy, but this is not true. Modern control valve design and sizing methods allow for the use of pumps with smaller head pressures, resulting in less energy absorption via the pressure drop across a control valve (Figure 2). A better-suited pump characteristic with a decreasing pressure p₁ at a decreasing flowrate Q would mean a smaller pressure drop (p₁–p₂) across the flowrate. Ideally, the pressure drop would be constant across the entire range. Pump manufacturers can only counteract this disadvantage brought about by an “improper” pump characteristic by using a VFD (Variable Frequency Drive) or VSD (Variable Speed Drive) to reduce the pump speed. Inclusion of a variable drive to reduce the speed of an electric motor-driven pump is not an entirely efficient solution, as energy savings are converted into heat within the pump motor and variable frequency/voltage converter. The greater the variation for 100 percent motor speed, the greater the amount of energy expended to achieve the modification.

Systems utilizing VFD/VSD control design are efficient compared to a control valve when the pump is more than 30 percent oversized. Any device that is exceedingly oversized represents a poor process control system design and is an inefficient design in and of itself. A more accurate representation of the control valve is as a “passive” control system element that only dissipates the pressure energy provided by the pump and plant. In a way, the control valve can be viewed as a scapegoat for inefficient control system design.

Figure 3. Pneumatic actuator with fail-safe action (“fail-close”)

Energy & Balance of Control Valves

Control valves used in modern industrial processes usually consist of a valve in globe, butterfly, rotary plug or other style; a pneumatic actuator with fail-safe action in case the supply air fails; and a smart electronic positioner. In on/off valves, the positioner is often replaced by a solenoid valve. Increasingly, digital positioners are also used for ESD (emergency shutdown) applications.

Normally, the positioner is powered by a 4 to 20 mA or fieldbus signal. The set point w expresses the desired valve opening that the positioner causes the valve to move to by adapting the signal pressure in the actuator. To do so, the positioner uses the energy available in the compressed instrument air network, which is usually operated at pressures between 45 PSI and 90 PSI.

The control loop, e.g., for pressure or temperature, that constantly adapts the set point for the positioner is run on a higher level in the process control system. As a result, the following energy sources are utilized in the control valve:

Electric power for the positioner or solenoid valve
Pneumatic energy from the compressed air network
Pressure relief energy of the fluid flow

We will now look at these energies in more detail.

Electrical Power Consumption

The power consumption is very low. Due to explosion-protection requirements, the electrical current in HART and fieldbus devices is limited and thus negligible. Typically, the power consumption is lower than 0.15 W.

Pneumatic Energy Consumption

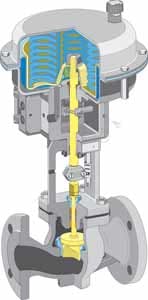
Figure 3 shows a typical example of a pneumatic actuator. The actuator is filled with air to compress the springs and lifts the actuator stem. As a result, the actuator takes air from the compressed air network that needs to be replenished by the compressor when the air is irreversibly vented to the atmosphere by the positioner the next time the actuator stem is extended.

If the set point w is changed and the valve position follows this preset exactly, the air volume provided by the compressed instrument air network in case of a step change from w_start to w_End is calculated as shown in the following equation. This is taking into account a rated valve travel H_Rated and an actuator with a bench pressure range from p_A0 to p_A100, an effective diaphragm area A_Eff and a dead volume V_A0 (normal air density r_N and reference pressure p_N = 1.0132 bar):

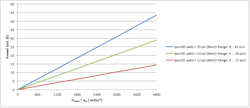
N_SZ means the number of switching cycles per year (1/a): The energy dissipation for 1 scfm is approximately 0.1275 kWh.¹ This would translate into annual costs of $76.80 (640 kWh). Figure 4 shows the annual costs to be expected for three spring range values pA100-pA0 vs H_Rated · A_Eff. It was assumed that the dead volume V_A0 accounts for approximately 10 percent of this volume. The product of the annual cycling rate (N_SZ) and stroke length (|w_Start-w_End|) was selected to be 100,000.

Figure 4. Costs of air consumption at (wEnd-wStart) NSZ = 100,000

This value was selected because it represented 100,000 cycles with 100 percent stroke length, as well as 10,000,000 cycles with 1 percent stroke length.

The air consumption of modern positioners is negligible, but required for operational safety. A typical value would be 3.53 scfh, which corresponds to approximately $2.10 (17.5 kWh) per year. In general, the air consumption when filling the actuator with air increases with:

Greater rated travel H_Rated
Greater amplitude of the step change w_End-w_Start
Greater effective actuator area A_Eff
Greater dead volume V_A0
Higher actuator rigidity expressed as p_A100-p_A0
Increased lower range value p_A0

The lower range value p_A0 increases with:

Greater flow force in the valve
Higher required shut-off force at the valve plug (leakage class)
Higher friction forces

The air flow used is considerably higher at high pressures in the network and with a large number of cycles. The air is increased during cycles when the actuator is completely vented in its closed position because the filling of the dead volume V_A0 was not taken into account in the equation mentioned above.

Hydraulic Energy Consumption

Energy is drawn from the fluid flow by the control valve’s throttling action. For liquids, the relation is simple:

The following applies to gases (k = adiabatic exponent, cr = pressure ratio for incipient speed of sound at the vena contracta, approximately 0.5 for globe valves):

Usually, the control valve intervenes by changing the flowrate within a fixed range between Q_min and Q_max. The smaller the pressure drop, the less energy is taken from the system. The system dependent, inconstant pressure drop caused by the opposing tendency of the pump and plant characteristics is detrimental in this case (Figure 2).

Figure 5 shows a comparison of performances at a pressure drop typically decreasing with the flowrate and at a constant pressure drop. The graph shows the disadvantage brought about by the improper pump characteristic.

Figure 5. Comparison of the valve’s throttling performances at fluctuating and constant p₁-p₂

The costs per kWh amounts to approximately $0.12. The resulting costs for hydraulic energy per year are around $1050 (8750 kWh) to $1320 (11,000 kWh), which is considerably higher than the costs incurred by the positioner’s power consumption and the air consumption.

Conditions & Actions to Reduce the Energy Consumption of Control Valves

The potential lies in optimized valve sizing, as well as in using highly efficient components. However, this also means that exact operating data without the usual high safety allowances must be available for sizing.

The following actions promise to yield the highest savings in energy consumption:

Selecting compact actuators, yet taking into account safety and control engineering aspects (actuator oversizing is indicated by an excessive ratio between the required and the actual actuator force);
Avoiding valves with oversized flow coefficients CV (an excessive CV allowance results in actuator oversizing);
Selecting valve accessories to match the demand (checking the required transit times and adapting boosters if applicable);
Using actuators with low leakage rates;
Avoiding high-friction pressure balancing components and stem seals;
Using smart positioners able to detect and indicate pneumatic leakage;
Optimizing the positioning loop (avoiding unstable control, e.g. overshooting or hunting); and
Optimizing higher-level process control loops (in some cases, control valves are not automated or poorly tuned to their control task).

Using Smart Positioners to Monitor and Diagnose the Energy Consumption of Control Valves

Smart positioners are expected to autonomously collect and assess online data. The basic assessment should include status data on the entire control valve’s performance that take into account NAMUR Recommendation 107 (self-monitoring and diagnostics of field devices). The status data is to be transmitted through standardized communication protocols (HART, PROFIBUS-PA, FOUNDATION fieldbus) and integration tools (EDDL, FDT/DTM, and, in the future, also FDI) in accordance with NAMUR Recommendation 105 (specifications for integrating fieldbus devices in engineering tools for field devices).

Figure 6. Energy efficiency with dynamic behavior (Step Response Test) Figure 7. Step Response of an ideal control system

To monitor the valve performance, the complex structure of a control valve should be stored in the positioner memory. This involves the individual components, such as valve, actuator, positioner, and further accessories (solenoid valve, booster, etc.), as well as the operating data used for sizing, such as the supply pressure, differential pressure, flowrate, and so on.

Statistical Data

Today, digital positioners can record statistical data—i.e., the essential operating data of the positioner—online. Basically, these are the set point w, the valve position x and the steady-state error e = w – x. This allows fundamental assessments, such as histograms and cycle counters, to be effectively performed.

Histograms of the set point w or the valve position x provide information on the position ranges the valve typically operates in. If the histogram shows that a control valve has only been operating between 30 percent and 50 percent over a longer period of time, the throttling effect actually needed is higher than expected and the valve’s Cv coefficient is oversized. The most probable cause for this is that the plant loss was expected to be higher and a pump with an excessive head was selected. The same flowrates could be achieved at a lower pressure drop if the pump did not feed too much pressure energy into the system and the plant loss calculations had not been based on excessive safety allowances.

Nevertheless, an oversized Cv coefficient requires a larger seat bore, which causes the actuator to be oversized as well. As a result, a greater air volume must be used, which increases the pneumatic energy consumption.

The equation mentioned in the previous section to calculate the air consumption for a step change of the set point is directly proportional to the step height. The more cycles the higher the resulting air consumption. Information on the current conditions is provided by the cycle counter running in the positioner and the histogram for the steady-state error e.

The cycle counter histogram indicates the number of travel direction changes versus the height of the amplitude. Values recorded in the error e histogram should mainly be in the range between -1 percent and 1 percent. If values occur outside this band, the positioner is frequently forced to reposition the valve, which consumes air.

When the set points are nearly constant and yet many valve travel cycles are recorded, positioning control is not optimal and disturbances (e.g., pressure fluctuations) considerably affect the process. Better procedures to test the control performance will be dealt with in the following section.

Specific Tests to Determine the Dynamic and Static Control Performance

Static performance refers to the steady-state accuracy, which can be negatively affected by excessive dead band and hysteresis values.

Static performance can be checked using the dead band and hysteresis test implemented in the positioner. During this test, the positioner internally changes the set point in very small steps (less than 1 percent) and records the value position after a defined waiting time. The set point and the resulting valve position are plotted in a diagram. The recorded points are connected within an adjustable set point range. When the upper range value has been reached, the positioner moves back to the lower range value in the same way. The distance between the two nearly parallel curves should be minimal.

Dynamic performance would be ideal if the valve position followed the set point given by the process control system exactly. In reality, however, this cannot be the case as the valve position always follows the set point with a certain delay. Additionally, the valve can overshoot and continue to oscillate for a short while until finally settling in the desired position. Where the energy consumption is concerned, the air to be supplied to the actuator when filling it to the upper bench range value is predetermined. Overshooting, however, causes unnecessary loss; as a result, overshooting should be minimized or avoided altogether.

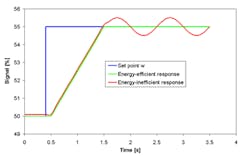
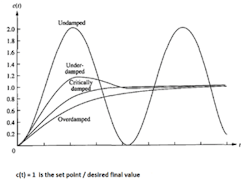
The dynamic performance can be checked using a step-response test (Figure 6). The selection of a step response test rather than the ramp or parabolic test correlates to a positioner’s purposes to achieve a constant position in steady-state. Without having to be triggered, a data logger integrated into the positioner records set point and valve position over time.

This makes the step response of the positioner visible in online operation. That response could be undampened, under-dampened, critically dampened, or overdampened. (Figure 7). The ideal step-response would be critically dampened as this represents the fastest response without overshoot. An overdampened response would be acceptable as it did not overshoot, but it does not represent the ideal response time. An undampened response would be unstable, uncontrollable, and the system would ultimately fail.

A step-response test is not required to identify an undampened system as the constant oscillation upon an initial change in set point would expose the design faults. Last, an under-dampened system would be wasteful as energy not required is expended each time the response overshoots the desired set point value. Applying the principles of optimal control, a solution could be found using weighted function for energy loss (overshoot) based upon the constraint that the settling time is acceptable. (EDITOR’S NOTE: Discussion of optimal control theory is beyond the scope of this article. If you are interested in more detail, please contact the authors. Contact details provided at the end of the article.)

Detailed Analysis of the Actuator Pressures

State-of-the-art positioners not only record the set point and valve position, but also log internal signals, such as the current input signal of the i/p converter, the supply pressure, and the output pressure.

Two correlations between these signals and their variations are significant in their ability to define energy consumption:

The output pressure vs. the valve position (Figure 8)
The output pressure vs. the current input signal

These two correlations are to be considered as reference graph lines (R1, R2), as well as current actual values in certain sections of operation (A1, A2):

Reference Actuator Pressure Signature R1: Recording the pressure conditions in the pneumatic actuator, which are determined by the compression of the actuator springs, without a medium flowing through the valve.
Reference Converter Characteristic R2: Recording the pressure conditions in the pneumatic actuator depending on the i/p converter current.
Actual Actuator Pressure Signature A1: Recording the current pressure conditions in the pneumatic actuator during operation while a medium flows through the valve in order to compare to the reference actuator pressure signature.
Actual Converter Characteristic A2: Recording changes of the converter characteristic while the process is running with flow through the valve.

Figure 8. Output pressure at the positioner (actuator) vs with the valve opening (signature) evaluated.

This data analysis allows a number of parameters to be logged that are important to diagnostics and ultimately energy monitoring:

The bench set of the actuator;
Excessive friction caused by the stem seal or balancing seal;
The leakage rate in the pneumatic system consisting of actuator, fittings/tubing connections, etc. also as a reference to the maximum positioner filling performance;
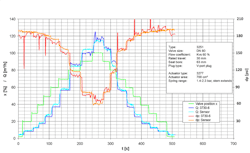
Using the pressure sensors in the positioner (Figure 9, SAMSON Type 3730 6 Positioner), the pressure drop that exists across the valve is determined indirectly based on a patented procedure;
A severely fluctuating differential pressure when the valve travels instead of an optimally constant value as low as possible (Figure 5); and
Flowrate through the valve (Figure 9).

Figure 9. Differential pressure and flowrate vs time when the travel changes; Q and Dp = p1-p2 measured by positioner sensor via indirect detection.

Figure 9 is based on a time-dependent measurement with the valve being opened in steps and then being closed again (green curve). The differential-pressure change is shown in the orange curve and the flowrate through the valve in the light blue curve. In the positioner, the internal pressure sensors indirectly detect the pressure drop across the valve using a patented procedure. The result is shown in the red curve. The congruence is sufficiently accurate. In addition, the flowrate Q can be estimated based on the Cv characteristic saved in the positioner memory. This is shown in the dark blue curve. Good estimation accuracy is achieved in this case as well.

Energy Monitoring & Assessment

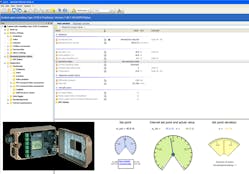
Based on the analyses mentioned in the previous sections, key performance indicators (KPIs) can be determined and visualized for the energy consumption as shown in Figure 10. These KPIs are:

The potential of saving energy from flow-related factors
The current throttling power of the valve
The air consumption (converted into electrical power or as an air volume)
The total electrical power consumption of the positioner

Exploiting all opportunities of energy-efficient valve sizing and selection with exact process data, allows a control valve to operate with the greatest energy efficiency. The valve will then be able to efficiently convert the required hydraulic power, which is determined by the pump and plant through an irreversible throttling process. Further energy efficiency is achievable through process control optimization of the valve positioning loop and the process control loop, which prevent overshooting and thus unnecessary air consumption. Finally, the addition of smart positioners can contribute to additional energy efficiency as smart positioners can monitor all energy-relevant KPIs while the process is running.

Figure 10. Energy-relevant key performance indicators of control valves determined by smart positioners (SAMSON Type 3730-6 Positioner) evaluated with SAMSON Trovis View.

As a result of energy-efficient control valve sizing and selection, process control optimization, and utilization of smart positioner monitoring of energy relevant KPIs; the design of an energy efficient process control system utilizing control valves is possible.

Dr. Jörg Kiesbauer, Domagoj Vnucec, Joseph Dufresne, and Jimmy Tsiantopoulos are representatives with Samson AG and Samson Controls, Inc. Samson offers a complete product line of instrumentation and controls valves, as well as modern integrated automation systems. Comments and questions about this article can be directed to Mr. Dufresne at [email protected] or 281 303-0117, ext. 524.

www.samson-usa.com

Reference

ZVEI brochure (German only), Mit Hightech für Umwelt und Klimaschutz, Der vielfältige Beitrag der Prozessautomation zur Energieeffizienz (High Tech for Environmental and Climate Protection; The Various Contributions of Process Automation to Energy Efficiency), June 2009.